When I say “standards-based” I mean that there should be a clear goal as to what the student is expected to learn.
I am a BIG believer that project-based learning can be great *IF* it is deliberately designed to be educational. I said that instead of standards-based because some people have a knee-jerk reaction to standards, “I hate Common Core!” Okay, whatevs.
When someone tells me that they are doing a math project, I want to see where the students are learning math and what specifically they are learning. If you have students filling in squares on graph paper, maybe they are learning about ratio and proportion but I’m a bit skeptical that your eight-year-old can now tell you that 2:4 is the same as 16:32 .
Here is a lesson plan for middle school students learning fractions.
Standards Taught
- Compare two fractions with different numerators and different denominators, e.g., by creating common denominators or numerators, or by comparing to a benchmark fraction such as 1/2
- Solve word problems involving addition and subtraction of fractions referring to the same whole, including cases of unlike denominators, e.g., by using visual fraction models or equations to represent the problem.
In theory, this is a standard that should be mastered in fourth grade. In practice, in most schools I have visited, students are learning fractions in fifth or sixth grade.
First, have students play Fish Lake.
You can download it here for free for Mac, Windows or iPad. We are making all of our games free as long as U.S. schools are closed.
Second, have students write their own math problems
After the student has played through the first level and come back to the camp, ask them to write their own math problem comparing fractions with different numerators and different denominators. Here is an example from a student at Tate Topa Tribal School.
Tasina and her mother both left the camp to pick berries this morning. They each walked for two hours, in opposite directions. At noon, they both turned around to come home. Tasina is 2/3 of the way back to the camp. Her mother is 3/4 of the way back. Who will get back first? How do you know this?
ANSWER: Her mother will get back first. I drew these out and 3/4 is longer.
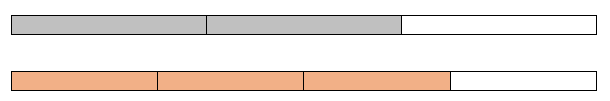
Third, have students answer and discuss each other’s problems
In the problem above, the student’s answer is correct and this is one way to look at it. It is perfectly fine to visualize a problem in this way.
Another way to answer this question is to change these to equivalent fractions with a common denominator.
An equivalent fraction to 2/3 is 8 / 12
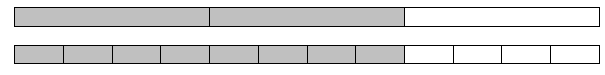
An equivalent fraction to 3/4 is 9/12
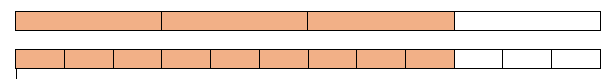
If you compare 8/12 and 9/12 it should be easy to see that 9/12 is more than 8/12.
What is wrong with the first way of solving this problem?
A student might ask what’s wrong with the way the student who wrote the problem answered it. My response to that is, “Absolutely nothing. There is absolutely nothing wrong with just drawing it out when you can.”
Visualizing a problem is a perfectly acceptable way to solve it.
The video discusses visualization, what it is, when it is and is not a good solution.
This same student had a second, more advanced, part to her problem, which you can find here.
